Information Theory: Applications to the Study of Mutation Dynamics
DOI:
https://doi.org/10.59973/emjsr.22Keywords:
Information theory, Information entropy (IE), Mass-energy, mass-energy-information, mutation dynamicsAbstract
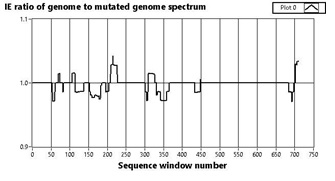
This study investigates the mutation dynamics of viral genomes using computational methods and information theory. The analysis focuses on Influenza-A virus genomes collected from Tianjin, China, between November 2009 and February 2011. The GENetic Information Entropy Spectra (GENIES) software is employed to calculate the information entropy (IE) of viral genomes and to compare them against a reference genome. The analysis reveals frequent mutation sites, with adenine (A) exhibiting the highest mutation frequency. The study provides valuable insights into the mutation patterns and dynamics of the analysed genomes, however, limitations in data size and the capabilities of the software are acknowledged, highlighting the need for further research and larger datasets to validate and expand upon these findings. Overall, this computational approach demonstrates the potential of using information theory and GENIES to enhance our understanding of viral mutation dynamics, with implications for vaccine design and preparedness for future viral strains.
References
P. M. Polgreen and E. L. Polgreen, Infectious Diseases, Weather, and Climate, Clinical Infectious Diseases, Volume 66, Issue 6, (2018), https://doi.org/10.1093/cid/cix1105 DOI: https://doi.org/10.1093/cid/cix1105
L. Herrero, E. Madzokere, What's the difference between mutations, variants and strains?, (2021),
https://www1.racgp.org.au/newsgp/clinical/what-s-the-difference-between-mutations-variants-a
M. Sobhanie, How do virus mutations happen, and what do they mean?, (2021),
https://wexnermedical.osu.edu/blog/virus-mutations-what-do-they-mean
R. Sanjuán, P. Domingo-Calap, Mechanisms of viral mutation. Cell. Mol. Life Sci. 73, (2016),
https://doi.org/10.1007/s00018-016-2299-6 DOI: https://doi.org/10.1007/s00018-016-2299-6
Cann, A. J. (2012). Genomes. Principles of Molecular Virology (Fifth Edition), Academic Press, (2016), DOI: https://doi.org/10.1016/B978-0-12-384939-7.10003-1
https://doi.org/10.1016/B978-0-12-801946-7.00003-1 DOI: https://doi.org/10.1016/B978-0-12-801946-7.00003-1
X. Li, M. Kong and J. Chen, et al., Epidemiology and full genome sequence analysis of H1N1pdm09 from Northeast China, (2013), https://doi.org/10.1007/s11262-013-0931-1 DOI: https://doi.org/10.1007/s11262-013-0931-1
R. V. L. Hartley, Bell System Technical Journal, 7: 3, Transmission of Information (1928),
https://doi.org/10.1002/j.1538-7305.1928.tb01236.x DOI: https://doi.org/10.1002/j.1538-7305.1928.tb01236.x
C.E. Shannon, A mathematical theory of communication, Bell Syst. Tech. J. 27 (1948), DOI: https://doi.org/10.1002/j.1538-7305.1948.tb00917.x
https://doi.org/10.1002/j.1538-7305.1948.tb01338.x DOI: https://doi.org/10.1002/j.1538-7305.1948.tb01338.x
J. A. Tenreiro Machado, "Shannon Entropy Analysis of the Genome Code", Mathematical Problems in Engineering, 2012, https://doi.org/10.1155/2012/132625 DOI: https://doi.org/10.1155/2012/132625
T. A. Reichert, D. N. Cohen and A. K. C. Wong, An application of information theory to genetic mutations and the matching of polypeptide sequences. Journal of Theoretical Biology. 42(2), (1973), https://doi.org/10.1016/0022-5193(73)90088-X DOI: https://doi.org/10.1016/0022-5193(73)90088-X
A. Veluchamy, et al., Information theoretic perspective on genome clustering. Saudi Journal of Biological Sciences. 28(3), (2021), https://doi.org/10.1016/j.sjbs.2020.12.039 DOI: https://doi.org/10.1016/j.sjbs.2020.12.039
C. Adami, The use of information theory in evolutionary biology. Annals of the New York Academy of Sciences, (2012), https://doi.org/10.1111/j.1749-6632.2011.06422.x DOI: https://doi.org/10.1111/j.1749-6632.2011.06422.x
M. M. Vopson and S. C. Robson, A new method to study genome mutations using the information entropy. Physica A: Statistical, (2021), https://doi.org/10.1016/j.physa.2021.126383 DOI: https://doi.org/10.1101/2021.05.27.445958
T. M. Nieuwenhuizen, The mass-energy-information equivalence principle. AIP Advances. 9(9), 095206, (2019), https://doi.org/10.1063/1.5123794 DOI: https://doi.org/10.1063/1.5123794
Downloads
Published
How to Cite
Issue
Section
License
Copyright (c) 2023 Clarence Marembo

This work is licensed under a Creative Commons Attribution 4.0 International License.