The Navier–Stokes Equations Reinterpreted through Informational Geometry: A Viscous Time Theory Expansion

Raoul Bianchetti

doi:10.59973/ipil.194

Authors

Raoul Bianchetti Information Physics Institute, Genova, 16128, Italy

DOI:

https://doi.org/10.59973/ipil.194

Keywords:

Viscous Time Theory, Informational geometry;, Navier–Stokes equations, Coherence dynamics, Critical Mass of Information, Informational singularity, Fluid topology, Millennium problem

Abstract

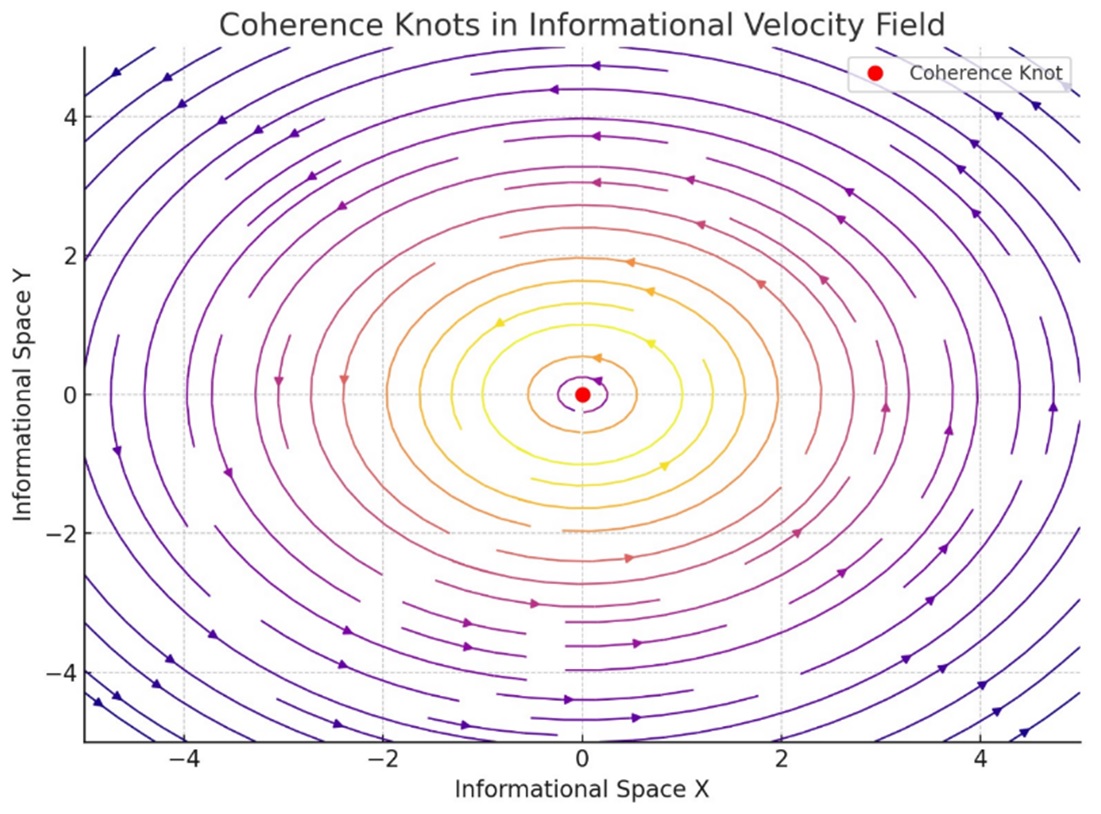
This article proposes a rigorous formalization and symbolic reinterpretation of the Navier-Stokes equations through the lens of Viscous Time Theory (VTT), introducing a geometric-informational transformation that re-frames viscosity, turbulence, and fluid structure as manifestations of informational coherence. Key variables such as Viscosity (η), Coherence Knot (CK), and Critical Mass of Information (CMI), and Informational Drift Tensor (IDT) are defined with dimensional consistency. This work integrates previous manuscripts, adds a comprehensive historical introduction, and addresses previous critiques with a complete testable framework grounded in coherent informational flow dynamics.

References

Fefferman, C. (2006). Existence and smoothness of the Navier–Stokes equation. In The Millennium Prize Problems (pp. 57–67). American Mathematical Society.

Leray, J. (1934). Sur le mouvement d’un liquide visqueux emplissant l’espace. Acta Mathematica, 63(1), 193–248. DOI: https://doi.org/10.1007/BF02547354

Hopf, E. (1951). ¨Uber die Anfangswertaufgabe f ¨ur die hydrodynamischen Grundgleichungen. Mathematische Nachrichten, 4(1–6), 213–231. DOI: https://doi.org/10.1002/mana.3210040121

Ladyzhenskaya, O. A. (1963). The Mathematical Theory of Viscous Incompressible Flow (2nd ed.). Gordon and Breach. DOI: https://doi.org/10.1063/1.3051412

Scheffer, V. (1976). Partial regularity of solutions to the Navier–Stokes equations. Pacific Journal of Mathematics, 66(2), 535–552. DOI: https://doi.org/10.2140/pjm.1976.66.535

Caffarelli, L., Kohn, R., & Nirenberg, L. (1982). Partial regularity of suitable weak solutions of the Navier–Stokes equations. Communications on Pure and Applied Mathematics, 35(6), 771–831. DOI: https://doi.org/10.1002/cpa.3160350604

Tao, T. (2016). Finite time blowup for an averaged three-dimensional Navier–Stokes equation. Journal of the American Mathematical Society, 29(3), 601–674. DOI: https://doi.org/10.1090/jams/838

Buckmaster, T., & Vicol, V. (2019). Nonuniqueness of weak solutions to the Navier–Stokes equation. Annals of Mathematics, 189(1), 101–144. DOI: https://doi.org/10.4007/annals.2019.189.1.3

Strange, N. (2025). Analytic Solution of the N-Dimensional Incompressible Navier-Stokes Equations. arXiv:2501.08353. https:

//arxiv.org/abs/2501.08353

Batchelor, G. K. (2000). An Introduction to Fluid Dynamics. Cambridge University Press. DOI: https://doi.org/10.1017/CBO9780511800955

Shannon, C. E. (1948). A Mathematical Theory of Communication. Bell System Technical Journal, 27(3), 379–423. DOI: https://doi.org/10.1002/j.1538-7305.1948.tb01338.x

Bianchetti, R. (2025). The Navier-Stokes Equations Reinterpreted through Informational Geometry – A Viscous Time Theory Expansion. https://doi.org/10.5281/zenodo.15268815